Third order system

Contents

Problem description

Time-optimal control of a third order system with bounded control.

Programmers: Gerard Van Willigenburg (Wageningen University) Willem De Koning (retired from Delft University of Technology)

% Copyright (c) 2009-2009 by Tomlab Optimization Inc.

Problem setup

% Array with consecutive number of collocation points
narr = [20 40];

toms t tf % Free final time

for n=narr

    p = tomPhase('p', t, 0, tf, n);
    setPhase(p)

    tomStates x1 x2 x3
    tomControls u1

    % Initial & terminal states
    xi = [0; 0.931; 0.9];
    xf = [2;     0;   0];

    % Initial guess
    if n==narr(1)
        x0 = {tf == 5; icollocate({x1 == xi(1); x2 == xi(2)
            x3 == xi(3)})
            collocate({u1 == 0})};
    else
        x0 = {tf == tfopt; icollocate({x1 == xopt1; x2 == xopt2
            x3 == xopt3})
            collocate({u1 == uopt1})};
    end

    % Box constraints
    cbox = {-1 <= collocate(u1) <= 1};

    % Boundary constraints
    cbnd = {initial({x1 == xi(1); x2 == xi(2); x3 == xi(3)})
        final({x1 == xf(1); x2 == xf(2); x3 == xf(3)})};

    % ODEs and path constraints
    dx1 = x2;
    dx2 = -x2-0.1*x2.*x2.*x2+x3;
    dx3 = -2*x3+-0.2*x3./sqrt(x3.*x3+1e-4)+2*u1;

    ceq = collocate({
        dot(x1) == dx1
        dot(x2) == dx2
        dot(x3) == dx3});

    % Objective
    objective = tf;

Solve the problem

    options = struct;
    options.name = 'Third order system';
    solution = ezsolve(objective, {cbox, cbnd, ceq}, x0, options);

    tfopt = subs(tf,solution);
    xopt1 = subs(x1,solution);
    xopt2 = subs(x2,solution);
    xopt3 = subs(x3,solution);
    uopt1 = subs(u1,solution);

Problem type appears to be: lpcon
===== * * * =================================================================== * * *
TOMLAB - Tomlab Optimization Inc. Development license  999001. Valid to 2010-02-05
=====================================================================================
Problem: ---  1: Third order system             f_k       2.956507317426365900
                                       sum(|constr|)      0.000000001016625562
                              f(x_k) + sum(|constr|)      2.956507318442991300
                                              f(x_0)      5.000000000000000000

Solver: snopt.  EXIT=0.  INFORM=1.
SNOPT 7.2-5 NLP code
Optimality conditions satisfied

FuncEv    1 ConstrEv   13 ConJacEv   13 Iter    9 MinorIter  111
CPU time: 0.046875 sec. Elapsed time: 0.047000 sec. 

Problem type appears to be: lpcon
===== * * * =================================================================== * * *
TOMLAB - Tomlab Optimization Inc. Development license  999001. Valid to 2010-02-05
=====================================================================================
Problem: ---  1: Third order system             f_k       2.949634637798716200
                                       sum(|constr|)      0.000001841571522688
                              f(x_k) + sum(|constr|)      2.949636479370239000
                                              f(x_0)      2.956507317426365900

Solver: snopt.  EXIT=0.  INFORM=1.
SNOPT 7.2-5 NLP code
Optimality conditions satisfied

FuncEv    1 ConstrEv    5 ConJacEv    5 Iter    4 MinorIter  133
CPU time: 0.062500 sec. Elapsed time: 0.062000 sec. 

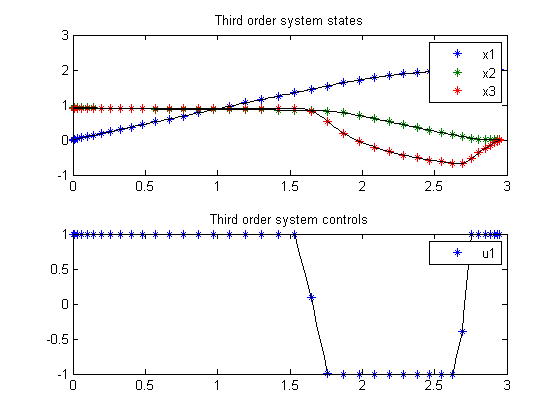
end

figure(1)
subplot(2,1,1);
ezplot([x1; x2; x3]); legend('x1','x2','x3');
title('Third order system states');

subplot(2,1,2);
ezplot(u1); legend('u1');
title('Third order system controls');